 |
 |
 |
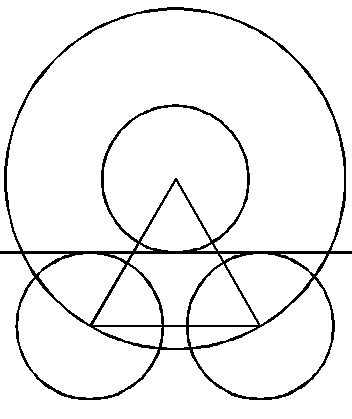 |
CROP CIRCLES AND QUANTUM MECHANICS
Many things have been said and written about the connection between crop circles, sound and music. Although this connection seems to be obvious at first sight, it turns out not to be plausible at all once we take a closer look at it!
If there is a connection, it is one with quantum mechanics, with super strings.
It's a connection with the nature of matter; not with music!
Indeed: a lot has been said and written about the presence of diatonic ratios in the crop circles' geometry. But let us take a closer look at diatonic ratios: what are they really?
When we look at the different notes on for instance a piano, we see that they relate to each other in a very specific way:
| First octave | 1 | 9/8 | 5/4 | 4/3 | 3/2 | 5/3 | 15/8 |
| Second octave | 2 | 9/4 | 5/2 | 8/3 | 3 | 10/3 | 15/4 |
| Third octave | 4 | 9/2 | 5 | 16/3 | 6 | 20/3 | 15/2 |
| Fourth octave | 8 | 9 | 10 | 32/3 | 12 | 40/3 | 15 |
| Piano notes | C | D | E | F | G | A | B |
These ratios create the harmonics in music and are therefore called diatonic ratios.
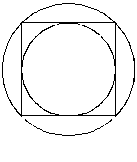
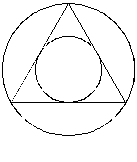
They can also be shown geometrically, as follows:
 |
 |
 |
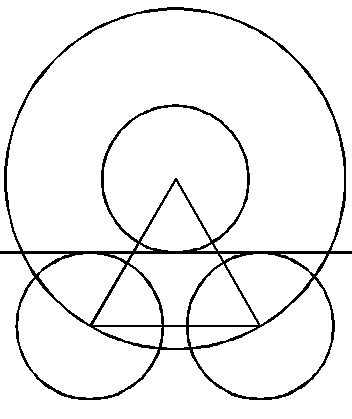 |
These geometrical ratios are quite often found in the geometry of crop circles. In my previous article I have shown that these ratios are a natural consequence of the type of construction technique used to create the crop circles. They are a logical part of the crop circles' geometry. As a result, the relationship "crop circles - music" seems to be obvious.
However there is another reason to assume that music - or at any rate sound - plays an important role in the crop circle phenomenon.
In order to understand this we have to take a closer look at the nature of sound.
Sound consists of vibrations that move forward by means of air pressure differences. Graphically it looks like this:
In other words, sound can be reproduced as a wave with peaks and lows. Sound that moves through air shows peaks in places with the highest pressure, and lows in places with the lowest pressure.
We can produce another tone that looks like this: When we produce both tones simultaneously, we get this picture:
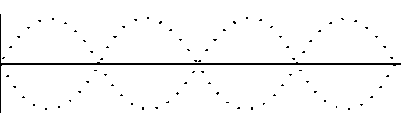
The waves in this graph can be added up. This is called interference: it is the way waves correspond to each other, the way they can be added up. In the above example the result is a horizontal line. The waves neutralise each other which results in no sound at all.
We can make this visible by taking melted paraffin and adding powder which will float through the paraffin. We will then add sound. The sound will move forward through the paraffin and the waves that arise this way will meet and interfere with each other. Together they will form extreme peaks and extreme lows, and there are also places where they will compensate each other. The latter happens when a peak meets a low: they extinguish each other. This interference process creates a pattern with spots that vibrate fiercely and spots that don't vibrate at all. An interference pattern arises. The powder will concentrate on the spots with the least vibration, by which it makes the interference pattern clearly visible for us!
The patterns that are created like this are often found in crop circles.
Does this mean there is a connection between music - or at least sound - and crop circles?
NO! This does not have to be a fact! It is even quite unlikely!
The above mentioned interference patterns arise with all sorts of waves, not just sound waves. Water waves show a similar pattern, just like radio waves. The interference pattern does not say anything about the type of wave involved. The only similarity between the patterns in the paraffin and the crop circles are the interference patterns! There are no indications whatsoever that the patterns in the crop are created by sound, or even that they refer to sound. The only possible indication is the presence of interference or rather the presence of interfering waves.
The chance that these are sound waves is minimal, since sound has a major drawback: it always needs a medium through which it can move forward. A medium that can assume and pass on the vibration of the sound source. Air is such a medium. If there is no medium sound can not move forward. On the moon - with its vacuum - we can hear no sound. In other words: sound is not a universal phenomenon!
This is not the case with Electro-magnetic waves, like for instance radio waves. These can indeed move forward without the help of a medium. They are indeed universal.
The only similarity between sound waves and Electro-magnetic waves is the ability to interfere. They can both cause interference patterns. Apart from that, these waves have absolutely nothing in common. To put it differently: interference patterns say absolutely nothing about the type of wave involved. They only say something about the interaction of waves. The paraffin experiments visualise this interaction. They show us a graphic reproduction of interference. A graphic reproduction with an unprecedented geometry! They show us that interference can be represented geometrically and that it has a strongly geometrical character. It shows the same geometry as we find in the crop formations.
But what about the diatonic ratios?
In order to understand their involvement we have to make a quantum leap, a leap into quantum mechanics. Quantum mechanics reached a height with the development of the Standard Model. All of the so far experimentally obtained data fitted perfectly in this model. Every experiment confirmed the Standard Model. The only problem was that the Standard Model was terribly ugly, complex and asymmetrical! And so, although everything seemed to confirm the Standard Model, something also seemed to be wrong! Furthermore, the deeper scientists went down to the depths of the subatomic world, the more they discovered strange, exotic particles!
For these problems concerning the Standard Model, scientists found a solution by developing the superstring theory.
When we make a string vibrate, we produce an audible tone. The pitch is determined by the string's length. A string of a certain length will produce a tone that is specific to that length, namely a tone with a frequency (=pitch) equal to the string's own vibration; a frequency in which the string will start to resonate. This own frequency depends on the length of the string. All of this enables us to play the violin!
Depending on the position of our fingers, we can play different notes. The note we hear is determined by the part of the string between our fingers and the bridge. By changing the distance between our fingers and the bridge, we play different notes.
Most essential here is the string, not the notes.
Analogue to this John Schwartz and Michael Green developed their superstring theory, with infinitely thin strings that can resonate in different pitches. These vibrations correspond with the various strings. Schwartz and Green believe that, if we magnify an elementary particle under a microscope - which unfortunately we cannot do yet - we would no longer see particles but vibrations: the vibrations of a superstring! The rest of the superstring theory was further elaborated by Edward Witten.
We can now deduce that it is not the elementary particles which are fundamental, but the harmonics created by the vibrating superstring. Matter therefore does not exist the way we experience it. Since we cannot magnify subatomic particles with our current techniques, we think of these particles as being elementary and fundamental. According to Schwartz, Green and Witten however, this is only appearance. According to them, the string and its vibrations are fundamental. Since a string is able to produce an infinite number of harmonics (just like the many notes we can play on a violin) we will observe an infinite number of particles.
The superstring theory can deduce the character of particles from the resonating vibrations of a string. It can also deduce Einstein's equations by stipulating the right conditions on space-time. In fact, all theories before the superstring's - like Einstein's, Kaluza's and the Standard Model - can be deduced from the superstring theory!
Conclusion
The geometry and forms that we find in crop circles are strongly related with the harmonics and interference patterns created by vibrating strings.
However there is no reason whatsoever to assume on this basis a connection with music or sound. If there is a connection at all, it is one of quantum mechanics. It's a reference to the nature of matter. Matter that does not exist the way we experience it in our day-to-day life. It's a reference to harmonics created by vibrating superstrings; the ones that give us the sensation of matter!
Copyright: Bert Janssen, 1998.

Return to the Crop Circle Connector